Time
by: G. J. W.
Imperial College of Science and Technology
Abstract:
This article
stresses the importance of observer dependent
timing as a consequence of the modern revision
of the concept of time.
Contents:
1.
The
Idea of
Time
a)
Evolution
of the Idea of
Time
b)
Concept
of Time in
Relativity
c)
Time,
Space-Time, and
Causality
d)
Time
and the Universe in Modern
Cosmology
2.
Measurement
and Determination of
Time
a)
Time
Scales
b)
Rotational
Time
(compare:
the
definition of
time
by The Order)
[source:
Americana Online:
time]
------------------------------------------------------------------------
Time. , The
fact that events take place and things have
duration in a dimension called "time" is
immediately familiar in human experience. Yet
the more deeply the idea of time is explored,
the more difficult it becomes. The way man
conceives time has changed over the centuries.
Today his understanding of time derives from the
most advanced fields of physics and astronomy.
The first part of this article reviews the
changing concepts of the nature of time. The
second part describes the ways in which time is
determined and measured.
1.
The
Idea of Time
One of the
peculiarities of modern civilization is the
importance it attaches to the idea of time. Time
is considered a kind of linear progression
measured by the clock and the calendar. The
tendency is to regard this idea as a necessity
of thought, but acquaintance with the beliefs of
other civilizations shows that this is not the
case.
a)
Evolution of the Idea of Time
Generally
speaking, time was not a concept of primary
importance in ancient thought. The Greeks tended
to regard the cosmic process as a cyclic
alternation of opposing forces rather than as a
continual evolution. For example, A. stated that
even the tendencies of heavy bodies to fall and
light bodies to rise are all part of a cyclic
process, so that these apparently straight-line
motions are in fact circular[Image]like
the perpetual circular motion of the heavenly
bodies, which had neither beginning nor
end.
A. also
held that time and motion were independent,
movement being measured by time and time by
movement. He recognized that time cannot cease,
whereas motion can[Image]except for
the
motion of the heavens
[Image]and
concluded that
time must be associated closely with this
motion, which he
regarded as the
perfect example of uniform motion.
Belief
in the cyclic nature of time was widespread in
antiquity,
and it gave rise to the idea of the Great Year.
There were two versions of this idea. In one,
the Great Year was considered simply as the
period required for the sun, moon, and planets
to attain the same positions relative to one
another as they had held at some previous given
time. This was the sense in which Plato used the
idea. On the other hand, the Great Year for H.
signified the period of the world from its
formation to its destruction and rebirth. These
two versions were combined by the Stoic
philosophers. The Stoics believed that when the
heavenly bodies returned to the same relative
positions they had at the beginning of the
world, everything would be restored as it was
before and the entire cycle would be renewed in
every detail.
Early
Christian
leaders rigorously
disputed
the traditional cyclical view of time. Prominent
among them was St. A. He
laid great emphasis on the idea that the
crucifixion of C. must be regarded as a unique
event, not subject to repetition, implying that
time is linear rather than cyclic. He was also
the pioneer of the
study of internal, or mental,
time.
Dissatisfied with A's close association of time
with motion, St A. chose to regard
time
as an activity of the
"soul," or
mind, endowed with powers of memory, attention,
and anticipation.
In the
scientific revolution of the 17th century, I. N.
took the view that time exists
independently
not only of human minds but also of all material
objects, and that it "flows" uniformly of its
own accord. On the other hand his contemporary
L., the German philosopher and mathematician,
regarded time
simply as the order
of
succession of phenomena.
The
problem of reconciling these different ideas of
time was tackled by the German philosopher I. K.
in the 18th century. K. was an enthusiastic
believer in N's natural philosophy, but he
rejected N's idea of time. Instead he argued
that time
is simply a feature of the way men's minds
visualize the external world
and is not
a characteristic of external reality itself. He
reasoned that if time were a characteristic of
the world, equally good arguments could be
advanced to show both that the world originated
and did not originate in time. In view of this
contradiction, K decided
that
time does not apply to the universe but only to
the way in which men think about the
universe.
b)
Concept of Time in Relativity
In the
19th century, as
a result of the arguments advanced by geological
and biological evolutionists, the modern idea of
time as linear advancement finally prevailed
over the older, cyclic conceptions.
[1]
The tempo of everyday life was speeded up, and
the temporal aspects of existence were
increasingly regarded as of predominating
importance. It therefore came as a shock when,
in 1905, A. E. pointed out an
unsuspected
difficulty in the prevailing idea of time and
concluded that time depends on the observer in a
way not previously
imagined,
even by K.
It had
been taken for granted[Image]both by
those who followed K's ideas and those who did
not[Image]that is a single worldwide
time order and that each instant of this order
corresponds
to a definite contemporaneous
state of
the whole universe. This, as Einstein observed,
was only an assumption. The order in which
events are perceived is not always the order in
which they are believed to occur. For a simple
example, lightning is seen before thunder is
heard, but both are manifestations of the same
electric discharge in the atmosphere.
Yet
until E. raised the question, it was universally
assumed as self-evident that when the rules are
found that determine the time of each perception
by the time of the event giving rise to it, all
events thus perceived must necessarily fall into
a definite time-sequence that is the same for
all observers.
After
much thought on the measurement of time and
motion, Einstein came to realize that a person
may have an immediate awareness of the
simultaneity of two events in his personal
experience but have no such awareness when one
event is directly experienced and the other
occurs at a distance. For example, suppose an
explosion were to occur on Mars. An observer on
earth records the instant at which he sees the
flash. If light traveled instantaneously, the
instant of observation on earth would coincide
precisely with the instant the explosion was
recorded by a hypothetical observer on Mars.
However, there is definite experimental evidence
that light takes time to travel, so the
terrestrial observer must in fact "correct" the
time recorded on his watch to make allowance for
the time taken by the light to reach
him.[2]
In
principle, the
velocity of light can be determined only if
the way to
measure time at all places that it traverses is
known. But this is precisely what
no
longer can be known, once the traditional
assumption of worldwide simultaneity for all
observers is
abandoned.
To escape this vicious circle, Einstein decided
to jettison the classical theory of the
measurement of space and time and begin on a
totally different basis.
The
classical theory, E. realized, leads to
absurdity when one tries to imagine what would
happen when traveling through space at the same
velocity as a beam of light. According to the
idea of relative motion based on classical
theory, the beam of light would then appear to
the observer to be at rest. And a vibrating
electromagnetic field at rest is a concept in
conflict with electromagnetic theory, which E.
saw no reason to reject. Instead
he
concluded that the laws of
physics[Image]including those concerning
the propagation of light[Image]must
remain the same for all observers in uniform
motion
[3],,
however fast they may be moving. In particular,
the velocity of light in empty space must be the
same for all such observers. Since this velocity
is finite,
it is the classical idea of relative motion that
must be modified.
A
curious result of E.'s theory is that, in
general, observers in relative motion must
assign different times to the same event
[4].
Only observers at relative rest can assign the
same time to the event. The discrepancy can be
illustrated by saying that a clock in relative
motion between two other clocks will measure a
smaller time interval than will the two clocks
at rest, as it passes from one clock to the
other. For velocities encountered in everyday
life the difference is negligible, but the
nearer the relative velocity of the moving clock
is to that of light, the greater the difference
will be. The relativistic effect has been amply
confirmed by experiments with elementary
particles moving at nearly the speed of
light.
c)
Time, Space-Time, and Causality
The
idea of the relativity of
time[Image]that is,
that
time depends on the observer and that there is
no absolute measure of
duration[Image]entails the relativity of
spatial measurement as well.
Due to the
universal character of the velocity of light,
the distance between two places can be measured
by the time it takes light to travel from one
place to the other. This measurement in turn
depends on the observer.
This
similarity between space and time is part of the
new universal concept introduced by the German
mathematician Hermann M. in
1908. If
an interval of time is regarded as a kind of
"distance" in the time dimension, it can be
converted into a distance by being multiplied by
c, the velocity of light, thus obtaining the
distance light would travel in that time. If the
time difference between any two events is T,
according to a particular observer, the
associated spatial interval is cT. Then if R is
the actual distance in space between these
events, it can be shown that although both cT
and R depend on the particular observer, the
difference between cT2 and
R2
has the same value
for all observers in uniform relative motion.
This difference is the square of what is called
the space-time interval between the two events.
Space-time is a four-dimensional analog of
three-dimensional space,
the
fourth dimension being the dimension of
time.
If the
universe is pictured as a system of events in
space-time, then the
times and spaces of different observers are
simply different "cross sections" or individual
perspectives of this system. [5]
Although the space-time interval between two
events is the same according to all observers at
rest or in uniform motion, it is split up by
different observers into different space and
time components.
The
idea of space-time also leads to the new concept
of forward and backward light-cones associated
with a given event E. Each of these cones has
the vertex E, and the cone surfaces are formed
by the space-time paths of all conceivable
electromagnetic rays passing through that
vertex. The forward cone is directed toward the
future; the backward cone converges toward E
from the past. Only those events lying inside
the forward or backward light-cone of a given
event E are in the future or past of that event
in any absolute sense. The temporal relationship
of all other events to E depends on the
observer. Thus for a given event F lying outside
the light-cones of E, observers can be found who
will regard F as later than E, simultaneous with
E, or earlier than E. No such ambiguity arises
in the classical theory of time, in which the
temporal relationship of any two events is the
same for all observers.
The
concept of light-cones has had a profound
influence on the concept of causality because
those events that lie inside each other's light
cones can be in absolute causal relationship
with one another. Einstein later extended his
ideas about space-time to include cases in which
observers are in accelerated motion in
gravitational fields.
d)
Time and the Universe in Modern
Cosmology
Although the classical concept of universal time
has been undermined, modern
cosmologists
have reintroduced the idea of a worldwide time
that is common to an important but restricted
class of observers.
According
to most cosmological theories,
there
is a preferential time scale at each place in
the universe.
This scale is associated with the "local" cosmic
standard of rest determined by the "local" bulk
of distribution of matter [Image]for
example, the center of mass of the stars in our
galaxy. The time scales of the observers
associated with these local standards of rest,
throughout the universe, "fit together" to form
one worldwide cosmic time.(note T.O.: compare
this to
the cakra tempometer-design at the
designspage)
It is
with reference to this cosmic time that
objective meaning can be given to concepts such
as the age of the earth, the sun, our galaxy,
and even the universe itself. Thus despite the
theory of relativity the concept of a cosmic
time scale can be retained, even though it is
not the time scale of every observer.
According to ideas based on the spectroscopic
study of light from distant galaxies, the
universe is believed to be expanding, which may
imply further limitations on the human idea of
time. If the rate of expansion is uniform, the
age of the universe would be about 10 billion
years, or about twice the age of the sun and the
earth. If the rate of expansion is decreasing,
the age would be somewhat less. However, if it
is increasing, not only would the age be
somewhat greater, but there also would be
regions receding from our own galaxy at
velocities greater than that of light. Hence no
light or any other physical influence from such
regions could ever reach our galaxy, and no
events in the regions could find a place in our
time scale. They would lie beyond our "time
horizon." Therefore no time scale could comprise
all events, and "worldwide" cosmic time would in
fact be restricted to events within each
observer's time
horizon.
See
also Relativity; Universe.
G. J.
W. Imperial College of Science and
Technology
Bibliography
A., A..,
Empires of Time: Calendars, Clocks, and Cultures
(Basic
Bks.
1989).
F., R., and M.
L., eds., The Nature of Time
(Blackwell
1987).
G., C. Social
Being and Time (Blackwell 1994).
H., M., The
Concept of Time, tr. by William McNeill
(Blackwell
1992).
Parallel German text and English
translation.
M., S. L.,
Time: A Bibliographic Guide (Garland
1991).
McI., P. K.,
Time and Experience (Temple Univ. Press
1991).
O., L. N., and
Q. S., The New Theory of Time (Yale
Univ. Press
1994).
S., Q.,
Language and Time (Oxford 1993).
T., S., and J.
G., The Discovery of Time (1965;
reprint,
Hippocrene Bks. 1983).
W., G. J., The
Natural Philosophy of Time, 2d ed. (Oxford
1980).
2.
Measurement and Determination of
Time
In
describing an occurrence, one must specify when
it took place and how long it lasted. Thus
timekeeping involves two concepts. Epoch
specifies the instant an event occurs, and time
interval specifies the time elapsed between two
events. Civil affairs, navigation, geodetic
surveying, and satellite tracking involve the
specifying of epoch, whereas physics involves
only time interval.
a)
Time Scales
The science of
time measurement is concerned with scales of
time to which events and time intervals can be
referred. Several kinds of scales are used to
meet different needs. Rotational time is based
on the rotation of the earth about its axis.
Ephemeris time is defined by the motion of the
earth about the sun and is used as the time
scale of celestial mechanics. Atomic time is
obtained from the operation of atomic
clocks.
Formerly rotational time, also known as mean
solar time, provided both epoch and the
fundamental unit of time interval, the second.
However, it was found that the rotational speed
of the earth is variable with respect to
ephemeris and atomic times, and so in 1956 the
mean solar second was replaced by the ephemeris
second. This in turn was replaced in 1967 by the
atomic second. The system of time measurement in
use is based on mean solar time for epoch,
whereas it is based on atomic time for
interval.
b)
Rotational Time
The
stars, sun, moon, and other celestial bodies are
located on an imaginary sphere called the
celestial sphere. As the earth rotates on its
axis the celestial sphere appears to rotate
about two fixed points, the north and south
celestial poles, at which extensions of the
earth's rotational axis would intersect the
celestial sphere. Rotational time is described
in terms of this sphere.
The
measurement of rotational time is based on the
angular position of celestial objects with
respect to the local celestial meridian of
longitude for a given observer. The local
celestial meridian is a great circle on the
celestial sphere, passing through the celestial
poles and the observer's zenith, or the point
directly overhead. The angular
distance[Image]that is, the
angle[Image]between the meridian and
another great circle passing through the
celestial poles and the object being observed is
called the hour angle of the object. It is given
a positive value when the object is west of the
meridian, and negative when it is east (see
diagram).[Image]
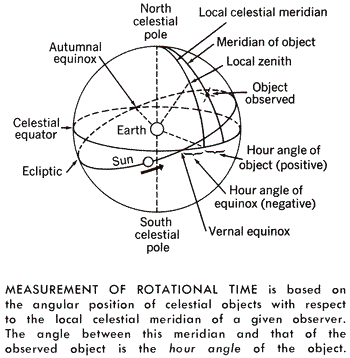
The
intersection of the plane of the earth's equator
with the celestial sphere is called the
celestial equator, and the intersection of the
plane of the earth's orbit with the celestial
sphere is called the ecliptic. The celestial
equator and ecliptic are inclined about
23[Image]27' to each other, and the two
points where they intersect are called the
equinoxes. One of these, called the vernal (or
spring) equinox, is a fundamental reference
point for the celestial coordinates of the
stars.
Go to
top of page.
Copyright © 1999 by Grolier Incorporated.
All rights reserved.
Notes
by The Order of Time:
[1] modern psychology reintroduced the
concept of cyclic time stressing the importance
of conditioning in recurring patterns of
time.
[2]:
think of our timesystem with timezones that give
the delusion of occurence at the same time while
in fact the sun rises in Berlin much earlier
than in Madrid.
[3}
Therefore in uniform motion time and space are
not independent of oneother and thus would the
theorem of non-cyclic linear time be rejected.
(practically: on the move in a train e.g. one
needs standardtime and staying home true timing
to the sun)
[4]
with the event of the sun, therefore
standardtime is at odds (makes a psychology or
instability of the time-experiece) with the
logics of physics in particular and science in
genral. compare time
as defined by The Order of Time
[5]
Compare with true time measurement relative to
standardtime measurement





